When it comes to teachers understanding fractions, it is a small world after all

As scarce as
international studies on teaching generally are, we were glad to see two new
studies looking at what it takes to be a successful math teacher. Both provide
more evidence that there are two distinct types of knowledge relevant to
teaching mathematics: content knowledge and pedagogical content knowledge. The
former is self-evident; the latter involves the capacity to understand possible
student misconceptions, to recognize alternative problem-solving approaches,
and so on. 

One study looks at teachers in Belgium (Teachers’ content and pedagogical content knowledge on rational numbers: A comparison of prospective elementary and lower secondary school teachers) while the other focuses on teachers in Taiwan and Germany (Content knowledge and pedagogical content knowledge in Taiwanese and German mathematics teachers). The studies share a few common and not wholly surprising findings:

The two types of knowledge are definitely
different and, fortunately, both can be measured.

The fact that secondary teachers have greater
content knowledge than lower level teachers doesn’t always imply that they have
greater pedagogical content knowledge.

Frankly, the
most interesting piece of information in the two articles is how poorly
Belgium’s elementary and lower secondary teachers performed on a test of
pedagogical content knowledge involving fraction problems (even though Belgian
students outperform ours both as 9-year olds and
15- year olds). It’s well established that American
elementary and middle school teachers are relatively weak in math, but it’s an
eye opener to see that on a math problem involving division by a fraction, 86
percent of teachers in higher-ranked Belgium were incorrect.

In any case,
this revelation of the difficulty elementary and middle school teachers have
with fractions no matter what side of the pond they’re on poses an excellent
opportunity to show very graphically the difference between two elementary math
textbooks we’ve evaluated and how each takes a very different approach to
developing both content knowledge and pedagogical content knowledge.

First a bit of math on the topic of division by
a fraction: Teachers should most definitely not reinforce the idea that the basis for this operation is
a mystery. (“Ours is not to wonder why, just invert and
multiply!”)  A good teacher can demonstrate the two types of situations
modeled by this equation:

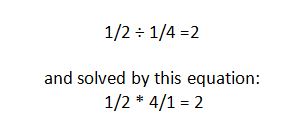
In the first
situation, we’re finding out how many quantities of 1/4th are in 1/2 (there are
2);  in the second situation, we’re
finding out that if we did 1/4th of a job with 1/2 of a quantity, it will take
2 of the quantity to complete the job.

(Keep
reading.  No one said this was easy!)

Here’s how a
textbook to which we gave a low score (Mathematics
for Elementary School Teachers, Bassarear, 5th ed.) presents this topic on p. 280:
There’s half a page of discussion that draws on the use of the multiplicative
inverse in an abstract way and provides no explanatory graphic. In fact, the
author explains, “Unlike most of the other algorithms we have examined,
[the invert-and-multiply algorithm] does not lend itself to a diagrammatic
representation.”

Here’s the final part of the explanation
offered:

Good thing
that Sybilla Beckmann (author of a textbook to which we gave a high score, Mathematics for Elementary Teachers with
Activities, 4th ed.) wasn’t paying attention to that conclusion. She
provides three pages of discussion demonstrating both types of situation
described above, with no fewer than four explanatory graphics—exactly the types
of graphics elementary teachers can use in their own instruction.

Here’s one
of the graphics (and
the full discussion can be found here):

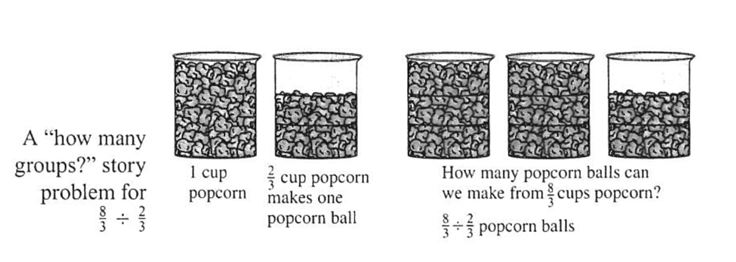
The quality
of textbooks in both elementary math and early reading prep vary dramatically.
Since our first reports on the preparation of elementary teachers in math
and reading, we’ve invested a lot in textbook reviews (analyzing the several
dozen elementary math textbooks and close to 1,000 early reading textbooks).
Ratings are based on extensive textbook reviews done by experts; in the case of
math textbooks, reviews are done by mathematicians well versed in the art of
teaching mathematically-skittish elementary teacher candidates. We’ve posted
both the math
and reading textbook reviews; doing more to ensure that teacher prep
instructors hear about these evaluations the next time they choose a textbook
for their course is critical.